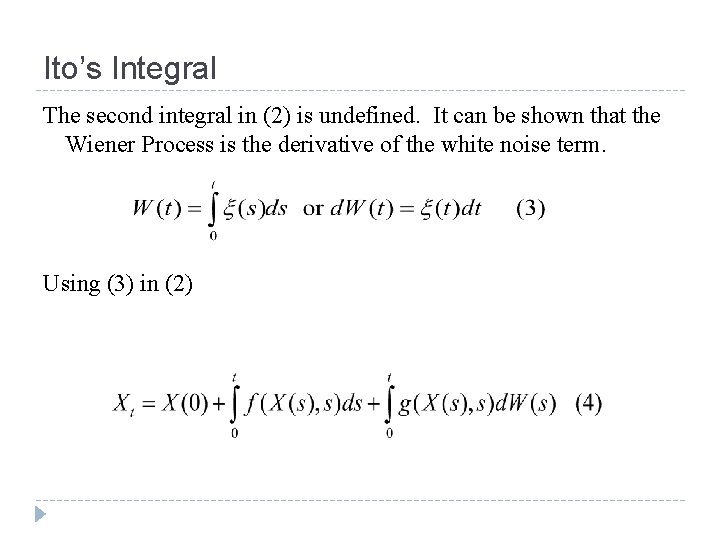
Ito Integral Of Exponential

Indefinite integrals Indefinite integrals are antiderivative functions. By the definition of a Wiener process if Δ t t i 1 t i then 1 Δ W t i 1 N 0 Δ t and 2 W t i is independent from Δ W t i 1.

The Logistic Growth Sde Motivation In Population Biology

Infty and there exists a partition.

Ito integral of exponential. DVleftdntimes pntimes dpright - its a usual full differential and its WRONG. In doing this the Integral Calculator has to respect the order of operations. 1 k du dx.

In other words there exists a sequence of real numbers c_k. ItV Z t 0 Vs dWs KX1 j0 jWtj1 tWtj t 2 Note. We then demonstrate Girsanovs change of measure formula in the case of general time scales.

4122021 Mean of exponential Ito integral. 0 1 by considering the Brownian increment Δ W t i 1 W t i 1 W t i. VtV0int_0tdVt Riemann would say that its easy.

Example 38 illustrates that the basic definition of Ito integrals is not very useful when we try to evaluate a given integral. Where k is any nonzero constant appears so often in the following set of problems we will find a formula for it now using u-substitution so that we dont have to do this simple process each time. 8312020 Expectation of exponential of Ito Integral 0 I need to calculate the covariance of two exponentials of Ito integrals where B is Brownian motion.

2192016 Our notation for a time integral of exponential Brownian motion is somewhat nonstandard. The multidimensional Itˆo Integral and the multidimensional Itoˆ Formula Eric Muller j June 1 2015 j Seminar on Stochastic Geometry and its applications. DVleftdntimes pntimes dpdntimes dpright.

2262021 Expectation of exponential of Ito Integral Asked 1 month ago by I need to calculate the covariance of two exponentials of Ito integrals where B is Brownian motion. Mt expBt. Du k dx or.

We know that Itt 0 is a martingale thus EIt EI0 0. 2212014 Posted on February 21 2014 by Jonathan Mattingly Comments Off on BDG Inequality. List of integrals of exponential functions 1 List of integrals of exponential functions The following is a list of integrals of exponential functions.

Based on this Its formula we give a closed-form expression for stochastic exponential on general time scales. ForasimpleprocessV t 0 satisfyingequation1 define theIt. Let phi be deterministic elementary functions.

Our result is being applied to a Brownian motion on the quantum time scale. To show the normal distribution note that for any fixed λ R. It transforms it into a form that is better understandable by a computer namely a tree see figure below.

252014 In this problem we will show that the Ito integral of a deterministic function is a Gaussian Random Variable. Integrals involving only exponential functions f x e f x d x e f x displaystyle int fxefxdxefx e c x d x 1 c e c x displaystyle int ecxdxfrac 1cecx. K12dotsN so that sum_k1infty c_k2.

For those with a technical background the following section explains how the Integral Calculator works. The alternative notation ItV is commonly used in the literature and I will use it interchangeably withthe integralnotation If the Brownian path t 7Wt were of bounded variation then the definition 2. A constant the constant of integration may be added to the right.

561 e x d x e x C. A specialty in mathematical expressions is that. WTH did the last term dntimes dp come from.

We let Mt denote the simple exponential martingale. Consider I t defined by I tint_0t sigma somegadB somega where sigma is adapted and sigma tomega leq K for all t with probability one. Integrals of Exponential Functions Exponential functions can be integrated using the following formulas.

Stack Exchange network consists of 176 QA communities including Stack Overflow the largest most trusted online community for developers to learn share their knowledge and build their careers. TextCovleft textexpleftint_0t fudB_u right textexpleftint_0s fudB_u right right mathbbElefteIteIs right mathbbElefteIt rightmathbbElefteI right. Cov exp 0 t f u d B u exp 0 s f u d B u E e I t e I s E e I t E e I.

First a parser analyzes the mathematical function. Then the Ito integral It Z u 0 σudB u has the normal distribution N 0 Rt 0 σ2udu. Furthermore VarI t Rt 0 σ2udu by the Ito isometry.

This is similar to the situation for ordinary Riemann integrals where we do not use the basic definition but rather the fundamental theorem of calculus plus the chain rule in the explicit calculations. For a complete list of Integral functions please see the list of integrals. 452021 Because if we did then we could integrate again.

Let σt be a nonrandom function. 10182018 The exponential function y e x is its own derivative and its own integral. Thats where the It.

Ito Calculus Wikiwand

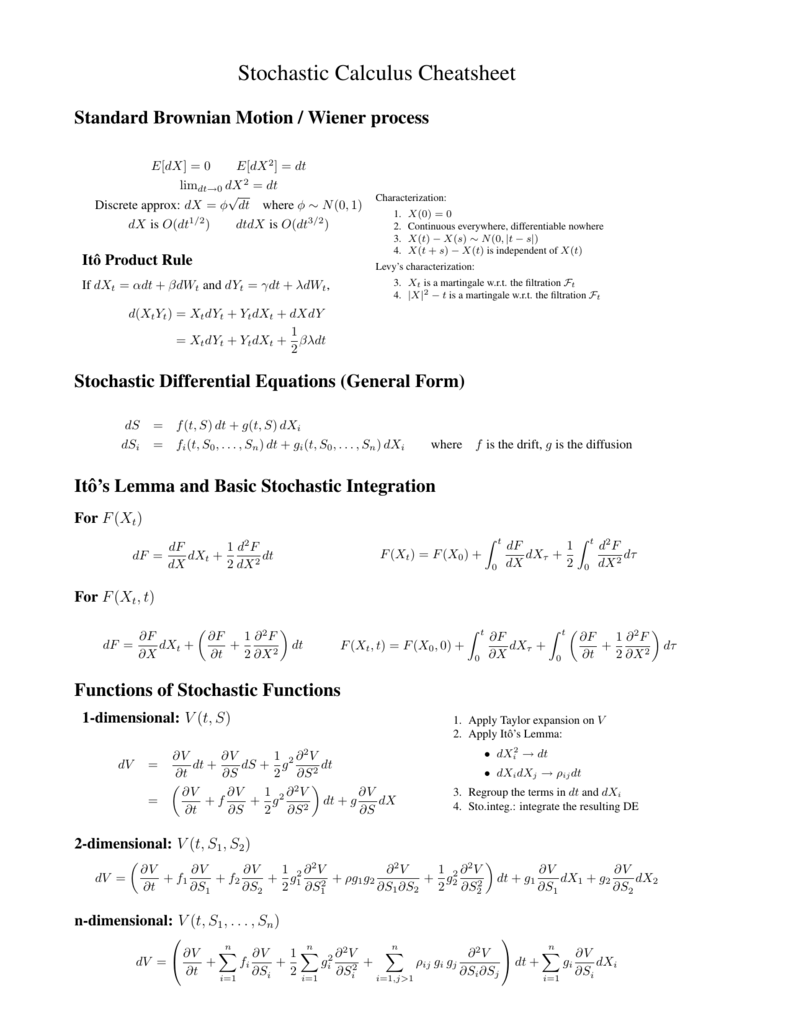
Stochastic Calculus Cheatsheet